Inclination functions

The inclination function may be considered similar to the simple inclination. It is applicable for vertical structures and it defines the horizontal inclination of the structure in the direction of global axes X and Y.

The inclination function is defined by means of an inclination-to-height curve. The curve is than assigned to the appropriate non-linear combination.

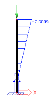
Let’s assume a single vertical column fixed at its foot. The column is subject to a vertical force.

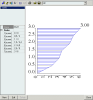
Let’s define an inclination curve in the Initial deformations manager.

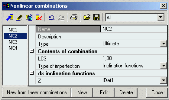
Let’s create a non-linear combination, adjust type of imperfection to Inclination function and select the previously defined curve.

The calculated horizontal displacement of the column will be affected by the imperfection.

It must be emphasised here that the inclination function is defined in absolute co-ordinates, i.e. in co-ordinates of the global co-ordinate system. Therefore, in order to successfully introduce an inclination defined by an initial deformation curve, the user must ensure that the curve is defined at the level corresponding to global Z co-ordinates of the structure – see the table below.

The curve of Initial deformation is defined as above.	The curve is defined for the interval <0 – 3.0> (measured along the global Z axis).
Variant A The column foot is in the origin of the global co-ordinate system, i.e. its vertical co-ordinate is equal to 0.0 (zero).	The result is affected by the inclination curve.
Variant B The column foot is in the point whose global vertical co-ordinate is equal to 3.0.	The result is NOT affected by the inclination curve as the curve definition ends just at the foot of the column and therefore the column is not subject to any inclination.