Seismic load

During earthquake, the subsoil (sub-grade or foundation) bearing a structure moves. The structure tries to follow this movement. As a result, all masses in the structure begin to move. Subsequently, they subject the structure to inertial forces. Supports can generally move in all directions, but normally only horizontal moves are taken into consideration. The user may define the direction that s/he considers to be crucial for the structure or s/he may evaluate the effect of shakes acting in different directions.

Inertial forces arise from the move. It is sufficient to determine these forces and apply them on the structure. Thus, the dynamic calculation is transformed into a static one. But the whole thing is not that simple. We do not know the precise movement of subsoil and therefore we are not able to determine the seismic forces precisely. But we can apply formulas of a technical standard or employ the frequency spectrum of a real earthquake.

Usually, horizontal movement of a structure is assumed for seismic load. That means that the earthquake acts in a plane horizontal to XY plane. The direction can be specified by means of coefficient for individual co-ordinate axes.

For example:

earthquake in X-direction	set X = 1 and Y = 0
earthquake in Y-direction	set X = 0 and Y = 1
earthquake in the axis of the 1st quadrant	set X = Y = 0.707 (i.e. sin(45°))

On the other hand, it is possible to take account of Z-directions as well. This can be achieved by specifying the coefficient for Z axis.

Note: We must be careful with the coefficients as earthquake "X=1; Y=0; Z=0.667" is not equal to earthquake "X=1; Y=0; Z=-0.667" nor to earthquake "X=-1; Y=0; Z=0.667".

The seismic calculation runs automatically, which means that both self-weight and input masses are used to generate load for individual eigenmodes.

The evaluation is performed separately for each force and displacement component using generally two available formulas:

Square root of the sum of squares taking account of the extreme value:

$image\formula_dyn1.gif$

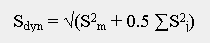
Square root of the sum of squares:

$image\formula_dyn2.gif$

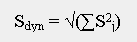
where:

Sdyn	component in consideration
Sm	the maximum corresponding component for individual eigenmode
Sj	other corresponding components for individual eigenmode

The final force may be both negative and positive. Both possibilities are considered in combinations.

Note : Whatever procedure we apply to the evaluation of quantity X, the result is always positive value. But we can have also a negative value because in seismicity the vibration is around the equilibrium position. The results of seismic calculation are always positive in SCIA Engineer. The only exception is with internal forces. Here, the co-ordinate system convention in not used. Instead, the "elasticity" convention (lower and front fibres under tension) is applied. Signs of some shear forces and bending moments may be inverted and "minus" may appear in the results of seismic calculation.

One more fact must be borne in mind. In static analysis we are curious about relations between individual internal forces – e.g. extreme axial force and corresponding bending moment. Such relations, however, cannot be determined for results of seismic calculation because each component is evaluated separately which, as you have surely noticed, is not a linear problem.

When evaluating results of seismic analysis, the one may say "this is the maximal axial force", "this is the maximal axial stress", "this is the maximal vertical displacement". But one cannot calculate stress in a section from the axial force and bending moment even though they appear in the same line of result table. This is the effect of the squares and roots in the formulas above. Accurate stress can be obtained only in appropriate module for design and checking (steel, concrete, etc. structures).